Mensaje de error

  • Deprecated function: The each() function is deprecated. This message will be suppressed on further calls en book_prev() (línea 775 de /home1/montes/public_html/drupal/modules/book/book.module).
  • Notice: Trying to access array offset on value of type int en element_children() (línea 6422 de /home1/montes/public_html/drupal/includes/common.inc).
  • Notice: Trying to access array offset on value of type int en element_children() (línea 6422 de /home1/montes/public_html/drupal/includes/common.inc).
  • Notice: Trying to access array offset on value of type int en element_children() (línea 6422 de /home1/montes/public_html/drupal/includes/common.inc).
  • Deprecated function: implode(): Passing glue string after array is deprecated. Swap the parameters en drupal_get_feeds() (línea 394 de /home1/montes/public_html/drupal/includes/common.inc).

Ejercicio 8.13 Hambley (Respuesta en Frecuencia, amplificador base comun)

Solapas principales

Enviado por pichu en Vie, 08/17/2012 - 22:32

a) Frecuencias de corte a alta frecuencia

Solucion:

\begin{displaymath}V_{CC=}\end{displaymath}


\begin{displaymath}R_{L}=R_{C}=510\,\Omega\end{displaymath}


\begin{displaymath}R_{S}=50\,\Omega\end{displaymath}


\begin{displaymath}R_{E}=1.3\,k\Omega\end{displaymath}


\begin{displaymath}C_{1}=C_{2}=1\,\mu F\end{displaymath}


\begin{displaymath}V_{CC}=15\,V\end{displaymath}


\begin{displaymath}-V_{EE}=-15\,V\end{displaymath}


\begin{displaymath}\beta=225\end{displaymath}


\begin{displaymath}0=R_{E}\frac{\beta+1}{\beta}\,I_{C}+V_{BE}-V_{EE}\end{displaymath}


\begin{displaymath}I_{C}=\frac{(V_{EE}-V_{BE})\,\beta}{\beta+1}=14.23\,mA\approx 10\,mA\end{displaymath}


\begin{displaymath}r_{o}=22.5\,k\Omega\end{displaymath}


\begin{displaymath}r_{x}=19\,\Omega\end{displaymath}


\begin{displaymath}r_{\pi}=585\,\Omega\end{displaymath}


\begin{displaymath}r_{\mu}=1.5\,M\Omega\end{displaymath}


\begin{displaymath}C_{\mu}=8\,pF\end{displaymath}


\begin{displaymath}C_{\pi}=196\,pF\end{displaymath}


\begin{displaymath}g_{m}=0.385\,S\end{displaymath}


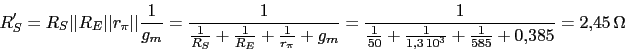
\begin{displaymath}R'_{S}=R_{S}\vert\vert R_{E}\vert\vert r_{\pi}\vert\vert\frac...<br /> ...ac{1}{50}+\frac{1}{1.3\,10^3}+\frac{1}{585}+0.385}=2.45\,\Omega\end{displaymath}


\begin{displaymath}f_{H_{1}}=\frac{1}{2\,\pi\,R'_{S}\,C_{\pi}}=\frac{1}{2\cdot \pi\cdot 2.45\cdot 196\,10^{-12}}=331.43\,MHz\end{displaymath}


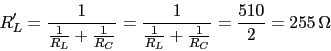
\begin{displaymath}R'_{L}=\frac{1}{\frac{1}{R_{L}}+\frac{1}{R_{C}}}=\frac{1}{\frac{1}{R_{L}}+\frac{1}{R_{C}}}=\frac{510}{2}=255\,\Omega\end{displaymath}


\begin{displaymath}f_{H_{2}}=\frac{1}{2\,\pi\,R'_{L}\,C_{\mu}}=\frac{1}{2\cdot \pi\cdot 255\cdot 8\,10^{-12}}=78.02\,MHz\end{displaymath}


b) Ganancia

Solucion:

\begin{displaymath}V_{CC=}\end{displaymath}


\begin{displaymath}R_{L}=R_{C}=510\,\Omega\end{displaymath}


\begin{displaymath}R_{S}=50\,\Omega\end{displaymath}


\begin{displaymath}R_{E}=1.3\,k\Omega\end{displaymath}


\begin{displaymath}C_{1}=C_{2}=1\,\mu F\end{displaymath}


\begin{displaymath}V_{CC}=15\,V\end{displaymath}


\begin{displaymath}-V_{EE}=-15\,V\end{displaymath}


\begin{displaymath}\beta=225\end{displaymath}


\begin{displaymath}0=R_{E}\frac{\beta+1}{\beta}\,I_{C}+V_{BE}-V_{EE}\end{displaymath}


\begin{displaymath}I_{C}=\frac{(V_{EE}-V_{BE})\,\beta}{\beta+1}=14.23\,mA\approx 10\,mA\end{displaymath}


\begin{displaymath}r_{o}=22.5\,k\Omega\end{displaymath}


\begin{displaymath}r_{x}=19\,\Omega\end{displaymath}


\begin{displaymath}r_{\pi}=585\,\Omega\end{displaymath}


\begin{displaymath}r_{\mu}=1.5\,M\Omega\end{displaymath}


\begin{displaymath}C_{\mu}=8\,pF\end{displaymath}


\begin{displaymath}C_{\pi}=196\,pF\end{displaymath}


\begin{displaymath}g_{m}=0.385\,S\end{displaymath}


\begin{displaymath}A_{vs}=A_{v}\,\frac{R_{i}}{R_{i}+R_{S}}\end{displaymath}


 

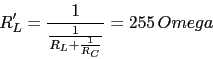
\begin{displaymath}R'_{L}=\frac{1}{\frac{1}{R_{L}+\frac{1}{R_{C}}}}=255\,Omega\end{displaymath}


\begin{displaymath}A_{v}=g_{m}\,R'_{L}=0.385\cdot 255=98.175\end{displaymath}


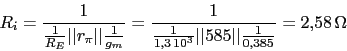
\begin{displaymath}R_{i}=\frac{1}{\frac{1}{R_{E}}\vert\vert r_{\pi}\vert\vert\fr...<br /> ...1.3\,10^3}\vert\vert 585\vert\vert\frac{1}{0.385}}=2.58\,\Omega\end{displaymath}


\begin{displaymath}A_{vs}=A_{v}\,\frac{R_{i}}{R_{i}+R_{S}}=98.175\,\frac{2.58}{2.58+50}=4.82\end{displaymath}


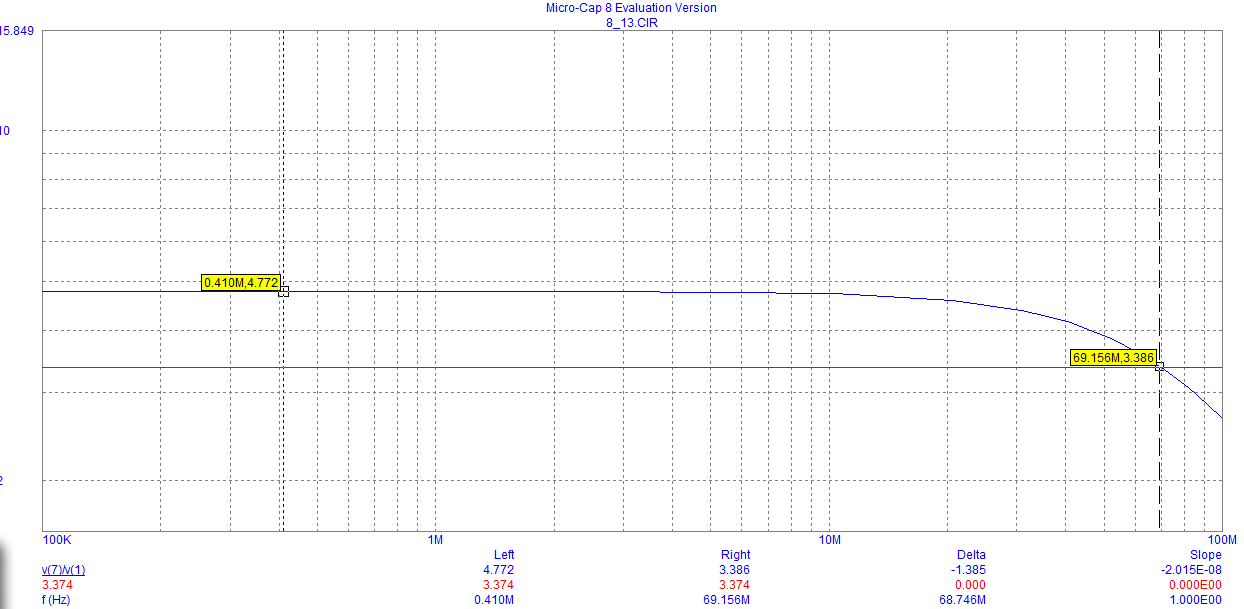
c) comprobar solucion con Spice, en este caso Micro-Cap

Solucion:

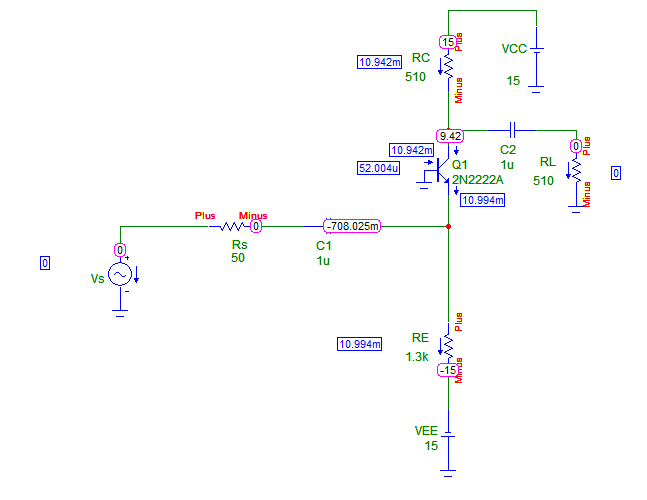
Image 8_13

Hacemos un AC analysis de la ganancia v(7)/v(1):

 

Image 8_13b

Tags:

Español

Añadir nuevo comentario

Más información sobre los formatos de texto

Plain text

  • No se permiten etiquetas HTML.
  • Las direcciones de las páginas web y las de correo se convierten en enlaces automáticamente.
  • Saltos automáticos de líneas y de párrafos.
Pin It